demand and its elasticity
Price elasticity of demand (PED or Ed) is a measure used in economics to show the responsiveness, or elasticity, of the quantity demanded of a good or service to a change in its price. More precisely, it gives the percentage change in quantity demanded in response to a one percent change in price (holding constant all the other determinants of demand, such as income). It was devised byAlfred Marshall.
Price elasticities are almost always negative, although analysts tend to ignore the sign even though this can lead to ambiguity. Only goods which do not conform to the law of demand, such as Veblenand Giffen goods, have a positive PED. In general, the demand for a good is said to be inelastic (or relatively inelastic) when the PED is less than one (in absolute value): that is, changes in price have a relatively small effect on the quantity of the good demanded. The demand for a good is said to be elastic (or relatively elastic) when its PED is greater than one (in absolute value): that is, changes in price have a relatively large effect on the quantity of a good demanded.
Revenue is maximized when price is set so that the PED is exactly one. The PED of a good can also be used to predict the incidence (or "burden") of a tax on that good. Various research methods are used to determine price elasticity, including test markets, analysis of historical sales data and conjoint analysis.
Contents[hide] |
[edit]Definition
PED is a measure of responsiveness of the quantity of a good or service demanded to changes in its price.[1] The formula for the coefficient of price elasticity of demand for a good is:[2][3][4]
The above formula usually yields a negative value, due to the inverse nature of the relationship between price and quantity demanded, as described by the "law of demand".[3] For example, if the price increases by 5% and quantity demanded decreases by 5%, then the elasticity at the initial price and quantity = −5%/5% = −1. The only classes of goods which have a PED of greater than 0 areVeblen and Giffen goods.[5] Because the PED is negative for the vast majority of goods and services, however, economists often refer to price elasticity of demand as a positive value (i.e., in absolute value terms).[4]
This measure of elasticity is sometimes referred to as the own-price elasticity of demand for a good, i.e., the elasticity of demand with respect to the good's own price, in order to distinguish it from the elasticity of demand for that good with respect to the change in the price of some other good, i.e., a complementary or substitute good.[1] The latter type of elasticity measure is called a cross-price elasticity of demand.[6][7]
As the difference between the two prices or quantities increases, the accuracy of the PED given by the formula above decreases for a combination of two reasons. First, the PED for a good is not necessarily constant; as explained below, PED can vary at different points along the demand curve, due to its percentage nature.[8][9] Elasticity is not the same thing as the slope of the demand curve, which is dependent on the units used for both price and quantity.[10][11] Second, percentage changes are not symmetric; instead, the percentage change between any two values depends on which one is chosen as the starting value and which as the ending value. For example, if quantity demanded increases from 10 units to 15 units, the percentage change is 50%, i.e., (15 − 10) ÷ 10 (converted to a percentage). But if quantity demanded decreases from 15 units to 10 units, the percentage change is −33.3%, i.e., (10 − 15) ÷ 15.[12][13]
Two alternative elasticity measures avoid or minimise these shortcomings of the basic elasticity formula: point-price elasticity and arc elasticity.
[edit]Point-price elasticity
One way to avoid the accuracy problem described above is to minimise the difference between the starting and ending prices and quantities. This is the approach taken in the definition of point-priceelasticity, which uses differential calculus to calculate the elasticity for an infinitesimal change in price and quantity at any given point on the demand curve: [14]
In other words, it is equal to the absolute value of the first derivative of quantity with respect to price (dQd/dP) multiplied by the point's price (P) divided by its quantity (Qd).[15]
In terms of partial-differential calculus, point-price elasticity of demand can be defined as follows:[16] let  be the demand of goods
be the demand of goods  as a function of parameters price and wealth, and let
as a function of parameters price and wealth, and let  be the demand for good
be the demand for good  . The elasticity of demand for good
. The elasticity of demand for good  with respect to price pk is
with respect to price pk is
 be the demand of goods
be the demand of goods  as a function of parameters price and wealth, and let
as a function of parameters price and wealth, and let  be the demand for good
be the demand for good  . The elasticity of demand for good
. The elasticity of demand for good  with respect to price pk is
with respect to price pk isHowever, the point-price elasticity can be computed only if the formula for the demand function, Qd = f(P), is known so its derivative with respect to price, dQd / dP, can be determined.
[edit]Arc elasticity
A second solution to the asymmetry problem of having a PED dependent on which of the two given points on a demand curve is chosen as the "original" point and which as the "new" one is to compute the percentage change in P and Q relative to the average of the two prices and the average of the two quantities, rather than just the change relative to one point or the other. Loosely speaking, this gives an "average" elasticity for the section of the actual demand curve—i.e., the arc of the curve—between the two points. As a result, this measure is known as the arc elasticity, in this case with respect to the price of the good. The arc elasticity is defined mathematically as:[13][17][18]
This method for computing the price elasticity is also known as the "midpoints formula", because the average price and average quantity are the coordinates of the midpoint of the straight line between the two given points.[12][18] However, because this formula implicitly assumes the section of the demand curve between those points is linear, the greater the curvature of the actual demand curve is over that range, the worse this approximation of its elasticity will be.[17][19]
[edit]History
Together with the concept of an economic "elasticity" coefficient, Alfred Marshall is credited with defining PED ("elasticity of demand") in his book Principles of Economics, published in 1890.[20] He described it thus: "And we may say generally:— the elasticity (or responsiveness) of demand in a market is great or small according as the amount demanded increases much or little for a given fall in price, and diminishes much or little for a given rise in price".[21] He reasons this since "the only universal law as to a person's desire for a commodity is that it diminishes... but this diminution may be slow or rapid. If it is slow... a small fall in price will cause a comparatively large increase in his purchases. But if it is rapid, a small fall in price will cause only a very small increase in his purchases. In the former case... the elasticity of his wants, we may say, is great. In the latter case... the elasticity of his demand is small."[22] Mathematically, the Marshallian PED was based on a point-price definition, using differential calculus to calculate elasticities.[23]
[edit]Determinants
The overriding factor in determining PED is the willingness and ability of consumers after a price change to postpone immediate consumption decisions concerning the good and to search for substitutes ("wait and look").[24] A number of factors can thus affect the elasticity of demand for a good:[25]
- Availability of substitute goods: the more and closer the substitutes available, the higher the elasticity is likely to be, as people can easily switch from one good to another if an even minor price change is made;[25][26][27] There is a strong substitution effect.[28] If no close substitutes are available the substitution of effect will be small and the demand inelastic.[28]
- Breadth of definition of a good: the broader the definition of a good (or service), the lower the elasticity. For example, Company X's fish and chips would tend to have a relatively high elasticity of demand if a significant number of substitutes are available, whereas food in general would have an extremely low elasticity of demand because no substitutes exist.[29]
- Percentage of income: the higher the percentage of the consumer's income that the product's price represents, the higher the elasticity tends to be, as people will pay more attention when purchasing the good because of its cost;[25][26] The income effect is substantial.[30] When the goods represent only a negligible portion of the budget the income effect will be insignificant and demand inelastic,[30]
- Necessity: the more necessary a good is, the lower the elasticity, as people will attempt to buy it no matter the price, such as the case of insulin for those that need it.[10][26]
- Duration: for most goods, the longer a price change holds, the higher the elasticity is likely to be, as more and more consumers find they have the time and inclination to search for substitutes.[25][27] When fuel prices increase suddenly, for instance, consumers may still fill up their empty tanks in the short run, but when prices remain high over several years, more consumers will reduce their demand for fuel by switching to carpooling or public transportation, investing in vehicles with greater fuel economy or taking other measures.[26] This does not hold for consumer durables such as the cars themselves, however; eventually, it may become necessary for consumers to replace their present cars, so one would expect demand to be less elastic.[26]
- Brand loyalty: an attachment to a certain brand—either out of tradition or because of proprietary barriers—can override sensitivity to price changes, resulting in more inelastic demand.[29][31]
- Who pays: where the purchaser does not directly pay for the good they consume, such as with corporate expense accounts, demand is likely to be more inelastic.[31]
[edit]Interpreting values of price elasticity coefficients
Elasticities of demand are interpreted as follows:[10]
| Value | Descriptive Terms |
|---|---|
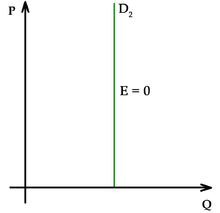
| Ed = 0 | Perfectly inelastic demand |
| - 1 < Ed < 0 | Inelastic or relatively inelastic demand |
| Ed = - 1 | Unit elastic, unit elasticity, unitary elasticity, or unitarily elastic demand |
| - ∞ < Ed < - 1 | Elastic or relatively elastic demand |
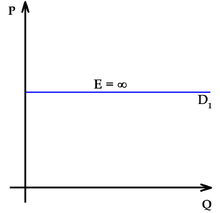
| Ed = - ∞ | Perfectly elastic demand |
A decrease in the price of a good normally results in an increase in the quantity demanded by consumers because of the law of demand, and conversely, quantity demanded decreases when price rises. As summarized in the table above, the PED for a good or service is referred to by different descriptive terms depending on whether the elasticity coefficient is greater than, equal to, or less than −1. That is, the demand for a good is called:
- relatively inelastic when the percentage change in quantity demanded is less than the percentage change in price (so that Ed > - 1);
- unit elastic, unit elasticity, unitary elasticity, or unitarily elastic demand when the percentage change in quantity demanded is equal to the percentage change in price (so that Ed = - 1); and
- relatively elastic when the percentage change in quantity demanded is greater than the percentage change in price (so that Ed < - 1).[10]
As the two accompanying diagrams show, perfectly elastic demand is represented graphically as a horizontal line, and perfectly inelastic demand as a vertical line. These are the only cases in which the PED and the slope of the demand curve (∆P/∆Q) are both constant, as well as the only cases in which the PED is determined solely by the slope of the demand curve (or more precisely, by the inverse of that slope).[10]
[edit]Effect on total revenue
See also: Total revenue test
A firm considering a price change must know what effect the change in price will have on total revenue. Revenue is simply the product of unit price times quantity:
- Revenue = PQd
Generally any change in price will have two effects:[32]
- the price effect : For inelastic goods, an increase in unit price will tend to increase revenue, while a decrease in price will tend to decrease revenue. (The effect is reversed for elastic goods.)
- the quantity effect : an increase in unit price will tend to lead to fewer units sold, while a decrease in unit price will tend to lead to more units sold.
For inelastic goods, because of the inverse nature of the relationship between price and quantity demanded (i.e., the law of demand), the two effects affect total revenue in opposite directions. But in determining whether to increase or decrease prices, a firm needs to know what the net effect will be. Elasticity provides the answer: The percentage change in total revenue is approximately equal to the percentage change in quantity demanded plus the percentage change in price. (One change will be positive, the other negative.)[33] The percentage change in quantity is related to the percentage change in price by elasticity: hence the percentage change in revenue can be calculated by knowing the elasticity and the percentage change in price alone.
- When the price elasticity of demand for a good is perfectly inelastic (Ed = 0), changes in the price do not affect the quantity demanded for the good; raising prices will cause total revenue to increase.
- When the price elasticity of demand for a good is relatively inelastic (-1 < Ed < 0), the percentage change in quantity demanded is smaller than that in price. Hence, when the price is raised, the total revenue rises, and vice versa.
- When the price elasticity of demand for a good is unit (or unitary) elastic (Ed = -1), the percentage change in quantity is equal to that in price, so a change in price will not affect total revenue.
- When the price elasticity of demand for a good is relatively elastic ( -∞ < Ed < -1), the percentage change in quantity demanded is greater than that in price. Hence, when the price is raised, the total revenue falls, and vice versa.
- When the price elasticity of demand for a good is perfectly elastic (Ed is − ∞), any increase in the price, no matter how small, will cause demand for the good to drop to zero. Hence, when the price is raised, the total revenue falls to zero.
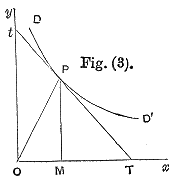
Hence, as the accompanying diagram shows, total revenue is maximized at the combination of price and quantity demanded where the elasticity of demand is unitary.[35]
It is important to realize that price-elasticity of demand is not necessarily constant over all price ranges. The linear demand curve in the accompanying diagram illustrates that changes in price also change the elasticity: the price elasticity is different at every point on the curve.
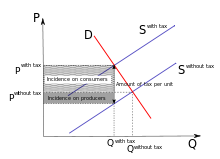
[edit]Effect on tax incidence
Main article: tax incidence
PEDs, in combination with price elasticity of supply (PES), can be used to assess where the incidence (or "burden") of a per-unit tax is falling or to predict where it will fall if the tax is imposed. For example, when demand is perfectly inelastic, by definition consumers have no alternative to purchasing the good or service if the price increases, so the quantity demanded would remain constant. Hence, suppliers can increase the price by the full amount of the tax, and the consumer would end up paying the entirety. In the opposite case, when demand is perfectly elastic, by definition consumers have an infinite ability to switch to alternatives if the price increases, so they would stop buying the good or service in question completely—quantity demanded would fall to zero. As a result, firms cannot pass on any part of the tax by raising prices, so they would be forced to pay all of it themselves.[36]
In practice, demand is likely to be only relatively elastic or relatively inelastic, that is, somewhere between the extreme cases of perfect elasticity or inelasticity. More generally, then, the higher the elasticity of demand compared to PES, the heavier the burden on producers; conversely, the more inelasticthe demand compared to PES, the heavier the burden on consumers. The general principle is that the party (i.e., consumers or producers) that has feweropportunities to avoid the tax by switching to alternatives will bear the greater proportion of the tax burden.[36]In the end the whole tax burden is carried by individual households since they are the ultimate owners of the means of production that the firm utilises (see Circular flow of income).
[edit]Selected price elasticities
Various research methods are used to calculate price elasticities in real life, including analysis of historic sales data, both public and private, and use of present-day surveys of customers' preferences to build up test markets capable of modelling such changes. Alternatively, conjoint analysis (a ranking of users' preferences which can then be statistically analysed) may be used.[37]
Though PEDs for most demand schedules vary depending on price, they can be modeled assuming constant elasticity.[38] Using this method, the PEDs for various goods—intended to act as examples of the theory described above—are as follows. For suggestions on why these goods and services may have the PED shown, see the above section on determinants of price elasticity.
|
Price Elasticity of Demand | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| In this chapter we look at the idea of elasticity of demand, in other words, how sensitive is the demand for a product to a change in the product’s own price. You will find that elasticity of demand is perhaps one of the most important concepts to understand in your AS economics course Defining elasticity of demand Ped measures the responsiveness of demand for a product following a change in its own price. The formula for calculating the co-efficient of elasticity of demand is: Percentage change in quantity demanded divided by the percentage change in price Since changes in price and quantity nearly always move in opposite directions, economists usually do not bother to put in the minus sign. We are concerned with the co-efficient of elasticity of demand. Understanding values for price elasticity of demand
Demand for rail services At peak times, the demand for rail transport becomes inelastic – and higher prices are charged by rail companies who can then achieve higher revenues and profits 
Wi-fi prices and price elasticity of demand From airports to hotels to conference centres. From inter-city rail services to sports stadiums and libraries, more and more people are demanding wireless internet connections for personal and business use. But demand is being constrained by the limited availability of services and, in places, high user charges. However the price of connecting to the internet through wi-fi services is set to fall as competition in the sector heats up. Nearly 90 per cent of laptops now come with wi-fi connections as standard and many public areas are being equipped with hotspots, but users often complain about the high price of accessing the internet. At present airports and hotels can charge high prices because in many cases a wi-fi service provider has exclusivity on the area. However the supply of wi-fi services is more competitive on the high street and prices are falling rapidly as restaurants and coffee shops are using low-priced wi-fi access as a means of attracting customers. The more wi-fi providers there are in the market-place, the higher is the price elasticity of demand for wi-fi connections. Wireless usage is growing across the UK with sales of 3G cards growing by 475%; these are mostly through business channels. In the consumer market, sales of Wi-Fi routers for the home have grown by 77% many broadband providers are now providing free wireless routers with each new broadband subscription. Note: WiFi stands for Wireless Fidelity Source: The Cloud and GFK UK Technology Barometer, 2006 Demand curves with different price elasticity of demand  Elasticity of demand and total revenue for a producer The relationship between price elasticity of demand and a firm’s total revenue is a very important one. The diagrams below show demand curves with different price elasticity and the effect of a change in the market price.  When demand is inelastic – a rise in price leads to a rise in total revenue – for example a 20% rise in price might cause demand to contract by only 5% (Ped = -0.25) When demand is elastic – a fall in price leads to a rise in total revenue - for example a 10% fall in price might cause demand to expand by only 25% (Ped = +2.5) The table below gives a simple example of the relationships between market prices; quantity demanded and total revenue for a supplier. As price falls, the total revenue initially increases, in our example the maximum revenue occurs at a price of £12 per unit when 520 units are sold giving total revenue of £6240.
Consider the price elasticity of demand of a price change from £20 per unit to £18 per unit. The % change in demand is 40% following a 10% change in price – giving an elasticity of demand of -4 (i.e. highly elastic). In this situation when demand is price elastic, a fall in price leads to higher total consumer spending / producer revenue Consider a price change further down the estimated demand curve – from £10 per unit to £8 per unit. The % change in demand = 13.3% following a 20% fall in price – giving a co-efficient of elasticity of – 0.665 (i.e. inelastic). A fall in price when demand is price inelastic leads to a reduction in total revenue.
Elasticity of demand and indirect taxation Many products are subject to indirect taxation imposed by the government. Good examples include the excise duty on cigarettes (cigarette taxes in the UK are among the highest in Europe) alcohol and fuels. Here we consider the effects of indirect taxes on a producers costs and the importance of price elasticity of demand in determining the effects of a tax on market price and quantity.  A tax increases the costs of a business causing an inward shift in the supply curve. The vertical distance between the pre-tax and the post-tax supply curve shows the tax per unit. With an indirect tax, the supplier may be able to pass on some or all of this tax onto the consumer through a higher price. This is known asshifting the burden of the tax and the ability of businesses to do this depends on the price elasticity of demand and supply. Consider the two charts above. In the left hand diagram, the demand curve is drawn as price elastic. The producer must absorb the majority of the tax itself (i.e. accept a lower profit margin on each unit sold). When demand is elastic, the effect of a tax is still to raise the price – but we see a bigger fall in equilibrium quantity. Output has fallen from Q to Q1 due to a contraction in demand. In the right hand diagram, demand is drawn as price inelastic (i.e. Ped <1 over most of the range of this demand curve) and therefore the producer is able to pass on most of the tax to the consumer through a higher price without losing too much in the way of sales. The price rises from P1 to P2 – but a large rise in price leads only to a small contraction in demand from Q1 to Q2. The usefulness of price elasticity for producers Firms can use price elasticity of demand (PED) estimates to predict: The effect of a change in price on the total revenue & expenditure on a product. The likely price volatility in a market following unexpected changes in supply – this is important for commodity producers who may suffer big price movements from time to time. The effect of a change in a government indirect tax on price and quantity demanded and also whether the business is able to pass on some or all of the tax onto the consumer. Information on the price elasticity of demand can be used by a business as part of a policy of price discrimination (also known as yield management). This is where a monopoly supplier decides to charge different prices for the same product to different segments of the market e.g. peak and off peak rail travel or yield management by many of our domestic and international airlines. Habitual spending on cigarettes remains high but sales are falling Sales of cigarettes are falling by the impact of higher taxes mean that smokers must spend more to finance their habits according to new research from the market analyst Mintel. Total sales of individual sticks for the UK in 2006 are forecast to be 68 billion, eleven billion lower than in 2001. Over a quarter of cigarettes are brought into the UK either duty free or through the black market. Total consumer spending on duty-paid cigarettes is likely to exceed £13 billion, 13% higher than in 2001. In the past, increases in the real value of duty (taxation) on cigarettes has had had little effect on demand from smokers because demand has been inelastic. But there are signs that a tipping point may have been reached. Sales of nicotine replacement therapies such as patches, lozenges and gums have boomed by nearly 50% over the past five years to around £97 million. But for every £1 spent on nicotine replacement, over £130 is spent on cigarette sticks. Nearly half of smokers tried to kick the habit last year. According to the Mintel research, smokers under the age of 34 are the most likely to stop smoking, with people aged 65 and over the least likely to try quitting. A ban on smoking in public places comes into force in England, Northern Ireland and Wales in the spring of 2007, the same ban became law in Scotland in March 2006. Sources: Adapted from Mintel Research, the Guardian and the Press Association  | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||
| Author: Geoff Riley, Eton College, September 2006 | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||
The Price Elasticity of Demand (commonly known as just price elasticity) measures the rate of response of quantity demanded due to a price change. The formula for the Price Elasticity of Demand (PEoD) is: PEoD = (% Change in Quantity Demanded)/(% Change in Price) Calculating the Price Elasticity of DemandYou may be asked the question "Given the following data, calculate the price elasticity of demand when the price changes from $9.00 to $10.00" Using the chart on the bottom of the page, I'll walk you through answering this question. (Your course may use the more complicated Arc Price Elasticity of Demand formula. If so you'll need to see the article on Arc Elasticity)First we'll need to find the data we need. We know that the original price is $9 and the new price is $10, so we have Price(OLD)=$9 and Price(NEW)=$10. From the chart we see that the quantity demanded when the price is $9 is 150 and when the price is $10 is 110. Since we're going from $9 to $10, we have QDemand(OLD)=150 and QDemand(NEW)=110, where "QDemand" is short for "Quantity Demanded". So we have: Price(OLD)=9 Price(NEW)=10 QDemand(OLD)=150 QDemand(NEW)=110 To calculate the price elasticity, we need to know what the percentage change in quantity demand is and what the percentage change in price is. It's best to calculate these one at a time. Calculating the Percentage Change in Quantity DemandedThe formula used to calculate the percentage change in quantity demanded is:[QDemand(NEW) - QDemand(OLD)] / QDemand(OLD) By filling in the values we wrote down, we get: [110 - 150] / 150 = (-40/150) = -0.2667 We note that % Change in Quantity Demanded = -0.2667 (We leave this in decimal terms. In percentage terms this would be -26.67%). Now we need to calculate the percentage change in price. Calculating the Percentage Change in PriceSimilar to before, the formula used to calculate the percentage change in price is:[Price(NEW) - Price(OLD)] / Price(OLD) By filling in the values we wrote down, we get: [10 - 9] / 9 = (1/9) = 0.1111 We have both the percentage change in quantity demand and the percentage change in price, so we can calculate the price elasticity of demand. Final Step of Calculating the Price Elasticity of DemandWe go back to our formula of:PEoD = (% Change in Quantity Demanded)/(% Change in Price) We can now fill in the two percentages in this equation using the figures we calculated earlier. PEoD = (-0.2667)/(0.1111) = -2.4005 When we analyze price elasticities we're concerned with their absolute value, so we ignore the negative value. We conclude that the price elasticity of demand when the price increases from $9 to $10 is 2.4005. How Do We Interpret the Price Elasticity of Demand?A good economist is not just interested in calculating numbers. The number is a means to an end; in the case of price elasticity of demand it is used to see how sensitive the demand for a good is to a price change. The higher the price elasticity, the more sensitive consumers are to price changes. A very high price elasticity suggests that when the price of a good goes up, consumers will buy a great deal less of it and when the price of that good goes down, consumers will buy a great deal more. A very low price elasticity implies just the opposite, that changes in price have little influence on demand.Often an assignment or a test will ask you a follow up question such as "Is the good price elastic or inelastic between $9 and $10". To answer that question, you use the following rule of thumb:
Data
Types of ElasticityMore Types of ElasticityMore on ElasticityRelated Articles Advertisement | |||||||||||||||||||||||||||||||||||||||||||||||||||||||||

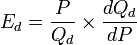







Comments
Post a Comment